Assistance for reducing to core CRM model

Posted by Christian Emil on 5/10/2017

The issue is resolved:

A super property (for all classes in crm and extensions)  is an extension in domain and range if the extra condition iff
A(x) & B(y) & P’(x,y) ⊃ P(x,y)​
​is true and  of course the standard ones
                               P (x,y) ⊃ A(x)
                               P(x,y) ⊃ B(y)
                               P(x,y) ⊃ P’(x,y)
​and
                               A(x)⊃ A'(x)
                               A(x) ⊃ B'(x)
                               P'(x,y) ⊃ A'(x)
                               P'(x,y) ⊃ B'(y)

Martin, Carlos and i have had a long email exchange on the topic, see a summary below. We  need an understandable syntax and a sort term for the phenomenon for the readers of the standard.  I suggest we put  an entry in the term listof CRM or extend the one for sub/super property.

Best,
Christian-Emil

To sum up what we seems to agree on: Assume, as you write, we study a part of the reality/world W and formulate a conceptualisation/ontology O for a given purpose. We then extend our focus from W to W’ and formulate an extension of O’ such that O’ union O is a conceptualization/ontology for W’.  Assume  in O P:A<->B. I O’ union O we extend P to P’ such that P:A’ <-> B’ where A’ and B’ are superclasses of A and B respectively in O’.

Martin: “We can regard the property P, originally declared in W, to extend into W' as you describe below. “

C-E: “Assume that P’:A’ <-> B’ in CRM. Assume extension where there A is a superclass of A’, B a superclass of B’  and  a property P:A<-> B  such that P’ is equal to P|A’ <-> P|B’. That is, as long as we restrict P to instances of A’ and B’ then P(x,y) iff P’(x,y).  Is the issue how to express the relation between P and P’ in FOL? ”

Martin: The point is, that we regard P not different from P' in its semantics, we only find out that it has a wider applicability, than anticipated restricting ourselves to W'.

As I understand the issue we need a syntax for restricting the subclass definition in FOL to ensure that P and P’ are identical when P’ is restricted to A and B:
Ordinary sub propery:
                               P (x,y) ⊃ A(x)
                               P(x,y) ⊃ B(y)
                               P(x,y) ⊃ P’(x,y)

The superproperty is an extension in domain and range otherwise it is the subproperty
                               P (x,y) ⊃ A(x)
                               P(x,y) ⊃ B(y)
                               P(x,y) ⊃ P’(x,y)
                               A(x) & B(y) & P’(x,y) ⊃ P(x,y)
 

In the 39th joined meeting of the CIDOC CRM SIG and ISO/TC46/SC4/WG9 and the 32nd FRBR - CIDOC CRM Harmonization meeting, CEO presented the solution and Martin the problem. Both of them  drew the following figures on the flipchart. 

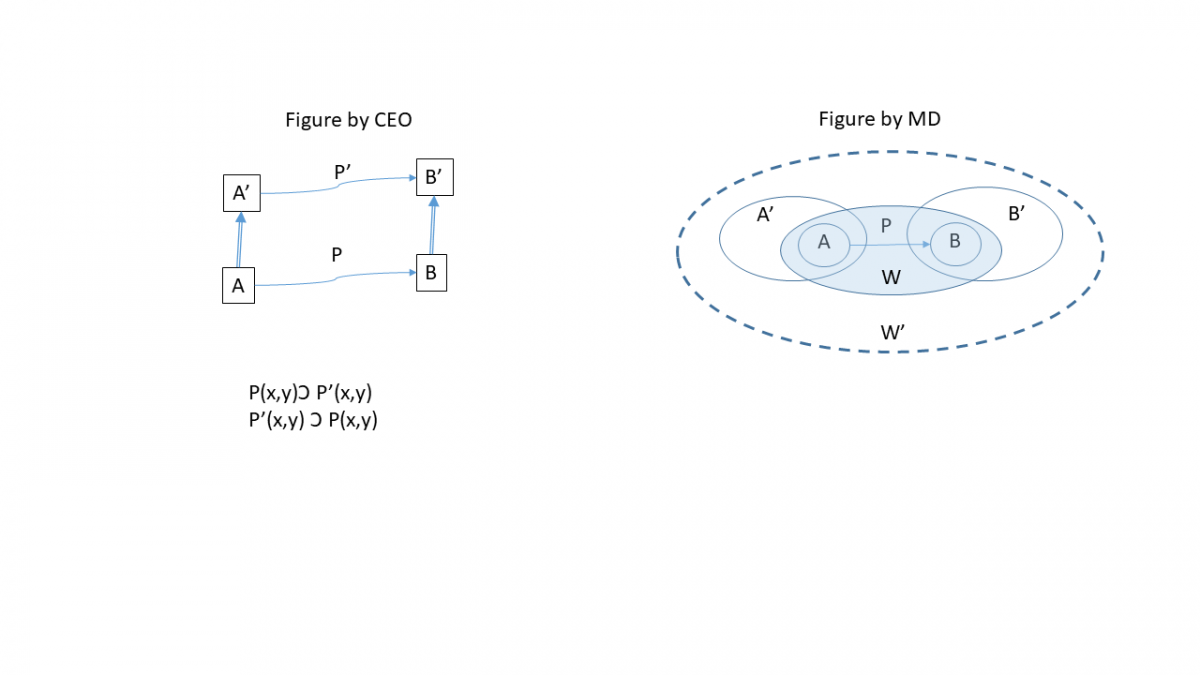
Then sig decided that we need a text and explanation diagram to be appear in super properties in terminology section. The text will be written by CEO, and Korina will make  the graphics  by the next meeting.

Iraklio, October 2017

posted by Christian Emil on 11/12018

<HW>

Dear all
Here is a first draft for the text. I realised that this is very similar to what is called conservative extention of a theory in logic.

Please comment and see if we can use the text as a draft.

Christian-Emil

**************************************************************************************************************************************************************

CRMbase (or an extention of it)  may be extended by declaring subclasses of existing classes as well as superclasses.   In the former case all properties of the CRM class will hold for the subclasses. In the latter case the scope CRMbase  will be extended and a property of the CRMbase class may hold for the new superclass but not necessarily. In the case  a property p of a class A also holds for a new superclass B it should be a conservative extension. That is, when restricted to the original class the extended property, p’, is identical to the original property p. In general  a superproperty is said to be a conservative extension of a subproperty when it is identical to the sub property when restricted to its domain an range.

Taken on its own, CRMbase is not affected by such an conservative  extension of scope, since it is not concerned with A. This is similar to what in logic is called a conservative extension of a theory. This construct is necessary for an effective modular management of ontologies, but is not possible with the current way RDF/OWL treats it.

In first order logic the conservative extension of a property can be expressed as follows. Assume that A and C are subclasses of B and D respectively and  that p, p’ are properties between A,C and B, D respectively:

                               A(x)  ⊃ B(x)
                               C(x)  ⊃ D(x)
                               P(x,y) ⊃ A(x)
                               P(x,y) ⊃ C(y)
                               P’(x,y) ⊃ B(x)
                               P’(x,y) ⊃ D(y)

If p’ is a conservative extention of p then

                               A(x) ∧ C(y) ∧ P’(x,y) ≡  P(x,y)

In the 41st joined meeting of the CIDOC CRM SIG and ISO/TC46/SC4/WG9 and the 34th FRBR - CIDOC CRM Harmonization meeting, the sig reviewed the text about the logical theory provided by CEO. This text describes the scope of CRMbase and that of the extensions in terms of logic (e.g., as sets). The text is here

The sig decided the following:

• The text is overall accepted, needs editing. This is assigned to  GB.
• The text should have an introduction of two phrases explaining the utility of this. This is assigned to Martin
• It should go on the end of the modelling extensions section of CRM document.

Lyon, May 2018​